The Fluoride Ion Selective Electrode Experiment
Direct
Potentiometry and Standard Addition Methods
Dr. David L. Zellmer
Revised February 15, 1999
The fluoride ion selective electrode experiment consists of the following parts:
In this method a series of fluoride standards are prepared in a background matrix of TISAB (Total Ionic Strength Adjustment Buffer). The unknown is prepared using TISAB in the hope that the matrix will be similar to the standards.
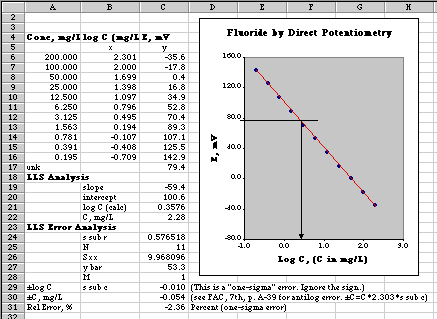
Since E = K + S log C
A plot of E vs. log C should yield a working curve that can be used to measure an unknown.

The first thing we learn from this plot is that our electrode is working properly, and has the expected linear response of E vs. Log C with slope measured at -59.4 mV, very near to the theoretical -59.2 mV at 25o C. A Linear Least Squares analysis of this plot allows us to measure the concentration of our unknown directly, and to compute the error of this determination. In the figure above the unknown was found to be 2.28 +or- 0.05 mg/L (one-sigma error).
Standard AdditionThe problem with direct potentiometry is the possible matrix effect on the unknown. The presence of iron(III), for example, might complex part of the fluoride in spite of the TISAB which is supposed to combat this. To check for this possibility we will need to use Standard Addition. [Note that the standard addition method described will not correct for the presence of other ions to which the electrode also responds; where E = K + S log(Cunk + kCintf)].
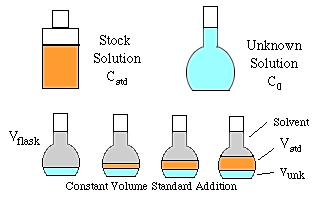
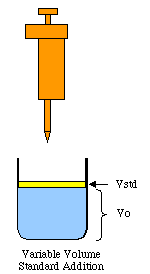
The graphics above show two types of standard addition: constant volume and variable volume. (For constant volume standard addition see the tutorial at www.csufresno.edu/chem/~davidz/Chem106/StdAddn/StdAddn.html).
The discussion below will be for variable volume standard addition. In this method we have a large volume of unknown to which small amounts of a standard solution are added. Although the total volume will not change a great deal, we will have to compensate for the volume changes produced. Note that a special pipet will have to be used that can dispense small volumes with high precision. (As an alternative, you could measure the density of the standard solution, then dispense the standard by mass using a weight buret.)
For an ion selective electrode with slope S that responds only to the analyte ion with concentration C,
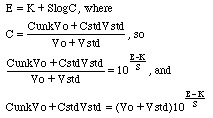
Now, if we wish to remove any effect that ties up part of the analyte ion, we need to divide the response with standard addition by the response without standard addition.
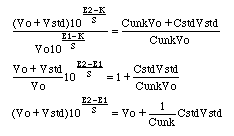
The final equation is in the form of y = mx + b, where y is the electrode response to the amount x of standard added. Note that x is not expressed as a concentration, but rather as the total amount CstdVstd of standard added. The slope of this line will be m=1/Cunk, from which we could compute Cunk directly. If we wish to use the more traditional standard addition approach using an x-axis intercept, then when y=0, the x-axis intercept will be at x=-b/m or CstdVstd=-CunkVo. Divide the amount CstdVstd at the x-axis intercept by Vo and you have Cunk.
Confused? The following example may help.
An unknown solution is prepared by working up a sample and buffering it with TISAB. 100.0 mL of this solution is placed in a beaker and its potential measured. This potential is called E1. Then 0.300 mL of a 300 mg/L standard is added, the solution mixed, and the potential measured. This potential is called E2. Additional standard is added, and additional values of E2 are measured. A plot of (Vo+Vstd)*10^((E2-E1)/S) is plotted vs. CstdVstd. The following graph was produced.
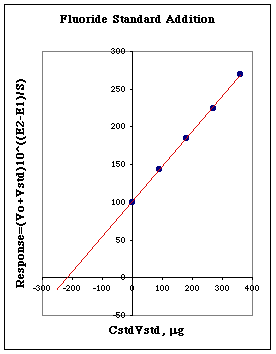
We can see that at y=0 a value of around 220 micrograms of fluoride is found. Since this was in 100 mL of solution the unknown must be around 220 micrograms/100 mL = 2.2 micrograms/mL or 2.2 mg/L. We are then told that the unknown solution was actually 2.15 mg/L, so we are pretty close.
Another important feature of this graph is the amount of increase shown in the response function for each addition of standard. Each amount of standard added should increase the fluoride level about 30% above the concentration of fluoride present due to the unknown. If the amount of standard added is too small, we will be left with a very large extrapolation to the x-axis. If the amount added is too large, the size of the x-axis intercept will be very small compared to the rest of the graph. Both of these extremes will produce unacceptable levels of relative error.
The spreadsheet that produced the graph above includes a careful linear least squares analysis of the results.
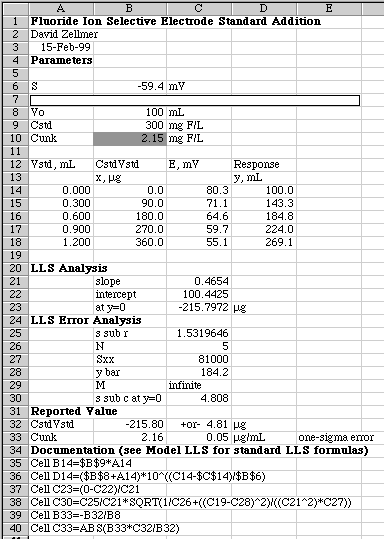
Some portions of this spreadsheet were blocked out because they represent "secret" knowledge about the unknown or about the response function of the electrode. The remaining numbers are similar to those found in a real experiment, however, and give a final result of 2.16 +or- 0.05 mg/L for the unknown solution. Compare this to the actual value of 2.15 mg/L, which is given as "secret" information in Cell B10. In a real analysis you would not have this value, of course.
Note that the value of "S" given in Cell B6 is the -59.4 mV found from the direct potentiometric method given above. Don't confuse this with the slope of the response function as computed by LLS analysis of the data from the standard addition runs. If a poor value of "S" is chosen, the Response function will become nonlinear, making it useless for a standard addition determination.
Note that the formula in Cell D14 is the Response function (Vo+Vstd)*10^((E2-E1)/S) which should be linear with respect to the number of micrograms of fluoride added to the system. When Vstd=0, the exponential term contains (E1-E1)=0 which makes the Response function equal to Vo or 100.0 mL. When filled down, the value of E1 stays fixed on Cell C14, while the value of E2 changes to the new potentials measured as each standard addition is made.
When doing an x-axis intercept at y=0, the value of M is assumed to be infinite because the value of y=0 is assumed to be perfectly known. The traditional formula for "s sub c" is modified by removing the 1/M term. The formula given in row 38 for Cell C30 contains an error which has confused a few people. The reference to cell C19 points to a blank cell. This happens to work out OK, since C19 refers to the y-value of the "unknown" which in this case of standard addition would be zero (the X-axis intercept is at y=0). The calculation comes out OK, since Excel assigned a value of zero to this blank cell. To correct the equation shown in row 38, replace "C19" with 0.
(See www.csufresno.edu/chem/~davidz/Stat/LLSTutorial/LLSmodel/LLSmodelSp99.html for details about the "traditional" LLS formulas.)
In Cell C33 the final one-sigma reported error for the unknown concentration is computed by multiplying the computed concentration of the unknown by the relative error of the x-axis intercept. The ABS() function is used to remove any minus signs that may result.
For current information, try websites for Corning and for Orion.
This page was last updated on May 18, 1999.
David L. Zellmer,
Ph.D.
Department of Chemistry
California State University,
Fresno